Model Description
This training aid demonstrates the translational and rotational kinematics of a rigid body in a translating and rotational frame. This demonstration should take 30 minutes.

Engineering Principle
Building upon general planar motion (GPM) in a non-rotational frame, this model develops the need to address GPM in a rotational frame by examining a projectile exiting a rotating gun tube of a moving tank. The fact that the projectile has its own velocity and that its distance from the tank changes with time motivates the fact that something is happening differently both physically and mathematically from the non-rotational previously discussed and leads to the derivation of the following vector equations:
What You Need
| Item | Quantity | Description/Clarification |
|---|---|---|
| Toy Tank | 1 | Ensure the turret swivels. |
How It’s Done
In Class: Tank clips start the lesson and the tank training aid is utilized to motivate the need for the derivation of the equations. The example problem for the lesson is then solved examining the absolute velocity and acceleration of the projectile before it leaves the tube.

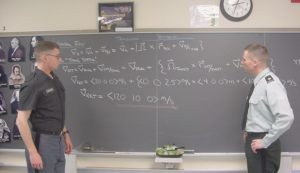
Additional Application: Tank Trivia – Did you know? Tanks have multiple targeting sensors which include a wind sensor on the back of the tank and a gyroscope which measures the angle of inclination or declination the tank is sitting or moving upon. When a gunner lazes a target, the computer measures the relative motion between the tank and its target, uses the wind and gyro, and instantaneously executes the targeting solution that will ensure steel target. The M1A2 Abrams also now includes independent targeting systems. This allows a Tank Commander (TC) to identify a target separate from the gunner such that when the gunner finishes a firing mission, a simple button automatically adjusts the turret to the secondary target.
