Model Description
This is a simple demonstration of the basic principles underlying the behavior of materials subjected to an axial load. The demonstration can also be used to show the usefulness of stress-strain curves versus load-deformation curves. This demonstration should take 10 minutes.

Engineering Principle
The basic definitions for deformation and strain:
where the axial (longitudinal) deformation is equal to the final length minus the initial length. The longitudinal strain is equal to the axial deformation divided by the initial length. Further, according to Hooke’s Law, the strain will follow the stress, so observed deformations are indicators of the magnitude of stress.
What You Need
| Item | Quantity | Description/Clarification |
|---|---|---|
| Bungee Cords | 3 | Make sure all three cords are the same length and width. Attach two cords together to make one long one. |
| Load Meter | 2 | A method of measuring the load to make sure that the same load is applied to each section of cord is required. |
| Tape Measure | 1 | It needs to be long enough to measure the total length when stretched. |
How It’s Done
Before Class: Prepare a method for hanging the bungee cords. Hooks above the blackboard are ideal, or hang them from a low ceiling or use another method.
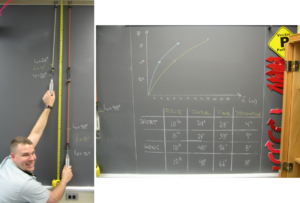
In Class: Hang the two different lengths of cords (figure above). Get an initial length of the cords (Put the load meters on just to put a little load on them to straighten them out). Apply an equal load to each of the cords and measure the final length. This allows you to calculate the deformation (figure below, left). Apply a greater load (doubling the initial load works well) and again measure the final length and calculate the deformation. Then plot the load deformation curve (figure below, right).
Observations: As is shown by the load-deformation curves, for every different length of material, you will need a separate curve. Then discuss how different thicknesses will also affect the results. This leads to the conclusion that load-deformation curves are very inefficient and cumbersome, hence the power of the stress-strain curve, which normalizes the load-deformation data for any size of the same material.
Additional Application: You can also drive home the point that load-deformation curves are inefficient by showing differences in deformation between bungee cords of the same length, but different thicknesses.
